The expected value of the payment of the exercise price is the exercise price times the probability of stock price exceeding exercise price (probability of exercise):ĭetermine the present value of the expected value of the payment by discounting this expected value using the risk free interest rate over the time remaining to the expiry of the option: Then calculate the expected value and present value of each component: Payment of Exercise Price and N(d2) Alternatively, when the stock price on the exercise date, S T, rises above the exercise price, X, i.e. This will only occur if the option is in the money. To understand this process, divide the payoff of the call option into 2 components:īoth of these are dependent on the option being exercised.
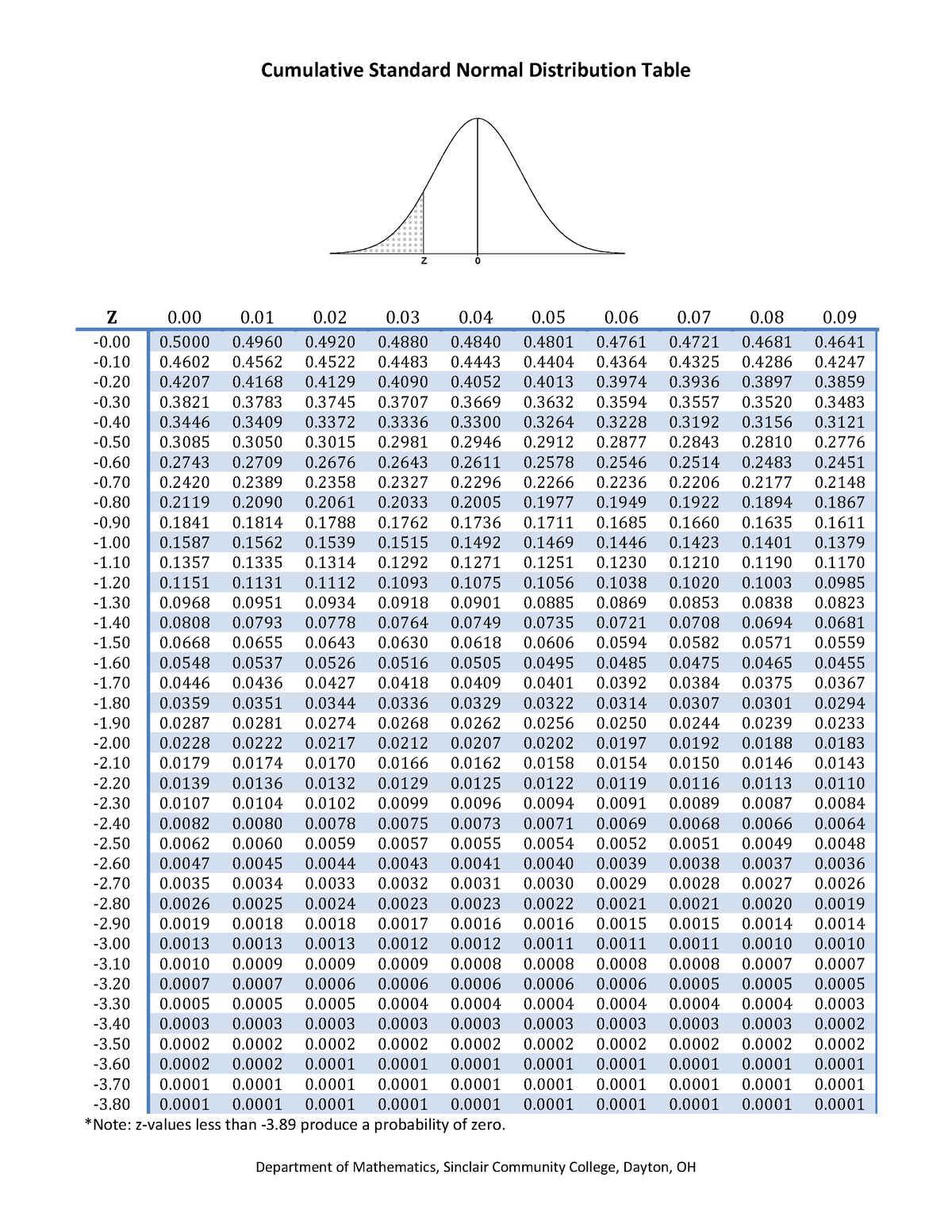
N(.) is the cumulative standard normal distribution, the risk adjusted probabilities.
S is the current value of the stock price Where C is the value of the European call option He does this by considering the value of European call option on a stock which pays no dividends prior to the expiry date of the option as given by the following formula: Lars Tyge Nielsen provides an interpretation of N(d 1) and N(d 2) and an explanation behind the difference between N(d1) and N(d2) under the Black Scholes Model.